Math Portal
Introductory Statistics
Section 5.2 - Probability Histrograms
A discrete random variable is one which may take on only a countable number of distinct values such as 0,1,2,3,4,........ Discrete random variables are usually (but not necessarily) something that is countable. If a random variable can take only a finite number of distinct values, then it must be discrete. Examples of discrete random variables include the number of children in a family, the Friday night attendance at a cinema, the number of patients in a doctor's practice, the number of defective light bulbs in a box of ten.
The probability distribution of a discrete random variable is a list of probabilities associated with each of its possible values. It is also sometimes called the probability function or the probability mass function.
Properties of the probabilities of a random variable:
Suppose a variable X can take the values 1, 2, 3, or 4. The probabilities associated with each outcome are described by the following table:
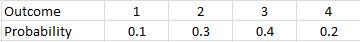

QUESTION 1: What is the probability that X is equal to 4?
P(X = 4) =
0.2
QUESTION 2: What is the probability that X is equal to 2 or 3?
P(X = 2 or X = 3) =
P(X = 2) + P(X = 3) =
0.3 + 0.4 =
0.7.
QUESTION 3: What is the probability that X is greater than 1?
P(X = 2) + P(X = 3) + P(X = 4)
0.3 + 0.4 + 0.2 =
0.9
A better way to answer this question is to use the Complement Rule.
1 - P(X = 1) =
1 - 0.1 =
0.9.
A discrete random variable is one which may take on only a countable number of distinct values such as 0,1,2,3,4,........ Discrete random variables are usually (but not necessarily) something that is countable. If a random variable can take only a finite number of distinct values, then it must be discrete. Examples of discrete random variables include the number of children in a family, the Friday night attendance at a cinema, the number of patients in a doctor's practice, the number of defective light bulbs in a box of ten.
The probability distribution of a discrete random variable is a list of probabilities associated with each of its possible values. It is also sometimes called the probability function or the probability mass function.
Properties of the probabilities of a random variable:
- 1. The probability of each value in the sample space of X must be between 0 and 1.
- 2. The sum of the probabilities of all of the values in the sample space of X is 1.
Suppose a variable X can take the values 1, 2, 3, or 4. The probabilities associated with each outcome are described by the following table:

QUESTION 1: What is the probability that X is equal to 4?
P(X = 4) =
0.2
QUESTION 2: What is the probability that X is equal to 2 or 3?
P(X = 2 or X = 3) =
P(X = 2) + P(X = 3) =
0.3 + 0.4 =
0.7.
QUESTION 3: What is the probability that X is greater than 1?
P(X = 2) + P(X = 3) + P(X = 4)
0.3 + 0.4 + 0.2 =
0.9
A better way to answer this question is to use the Complement Rule.
1 - P(X = 1) =
1 - 0.1 =
0.9.